How To Tormek etc., calculation of tool rest position for desired angle

[Mr.Wizard]

This has been done by others, and I don't even own a Tormek. However it was a good exercise to derive the formulas myself and I'd like to share one of the results as I find it pleasing: the formula for the gap (green line). After a bit more work I think I also have a simple form for the y formula.

gap =

y =

 

[Mr.Wizard]

For those who are not yet fans of the complex plane there is another equally simple if more verbose way to express this, and that is using vectors. We'll need to define a two argument function P that takes (magnitude, angle) and returns Cartesian components (x, y). (I avoid the standard r/theta to avoid confusion with their earlier use.)

P(m, a) = ( m cos(a), m sin(a) )

Then we can write:

|| (x, y) || is the norm, i.e. distance, i.e. sqrt( |x|^2 + |y|^2 ). All angles are in radians.

What this represents is defining the contact point on the wheel as (0, R) and then walking (d-r, theta) and (r+t, theta-90°) from that point for the composite tool depth and rest offset respectively, giving us the coordinates of center of the tool rest relative to the origin at the center of the wheel.

 

[sickpuppy1]

Wow, and here I was happy with a magic marker!!

 

[Diemaker]

Better get a set of big micrometers to measure wheel size!

 

[cbwx34]

Better get a set of big micrometers to measure wheel size!

Wheel size is not that critical... a simple metric ruler, or even the markings printed on the machine, will do the job.

Might also be worth mentioning that there are several calculators available, that do the work for you... several are listed HERE

 

[kreisler]

gap =

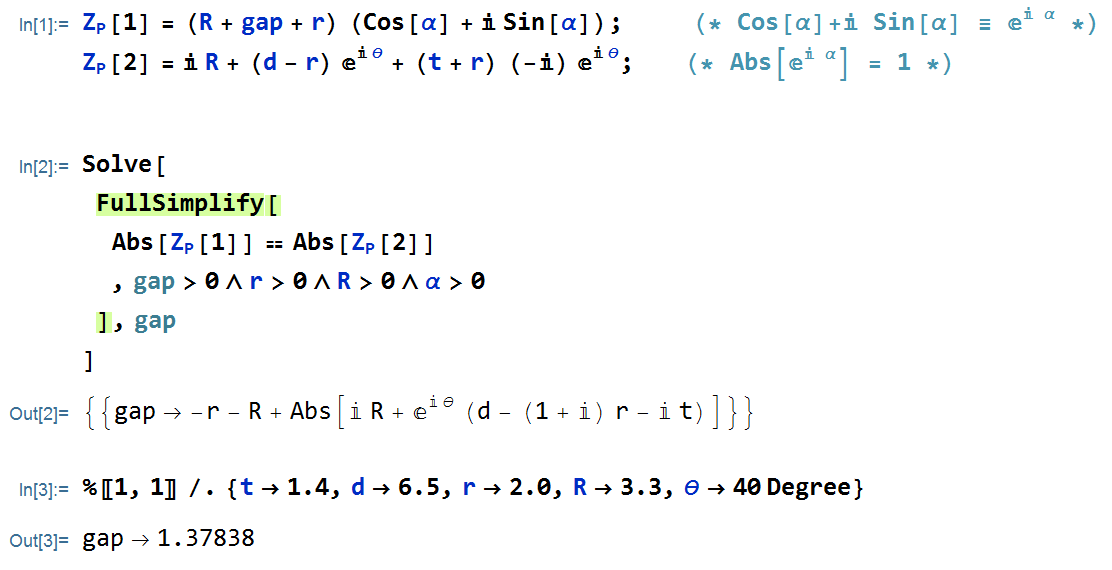
using plain/plane geometry i managed to setup three cosine formulas for 2 adjacent triangles, introducing 2 additional auxiliary variables and the software managed to solve the set of three nonlinear equations, and for a numerical example it produced the same result (gap=1.37838cm) but that was not the way to go, way too complicated. your approach M Mr.Wizard using the complex plane is the most elegant path, of course giving the same numerical result. i had to refresh my basic knowledge on complex pointers Z and then was able to restate your solution:

complex pointers are in many ways "the same" as position vectors in the 2D-plane, so your two "different" approaches are basically the same, unlike my idea of the Law Of Cosines never mind. anyway, given a set of parameters {t, d, R, r, 40°} it is still not very easy to operate the "simple" formula on a handheld calculator (-> user error ).

and me too, i doht have an original tormek either